🕑 Reading time: 1 minute
Continuous beams and one-ways slabs are statically indeterminate structures which i.e they cannot be analyzed using three equations of equilibrium developed to solve unknown moments and reactions of determinate members. That is why several methods such as slope deflection method, moment distribution method, displacement method and stiffness matrix, and ACI approximate method have been developed to analyze statically indeterminate structures.
The ACI approximate method, which is also called the simplified method of analysis, is presented in ACI 318-19, section 6.5 to analyze continuous beams and non-prestressed one-way slabs. The ACI approximate method is derived by elastic analysis, considering alternative placement of live load to produce maximum positive or negative moment at critical sections.
The approximate moments and shears, found by using ACI simplified method, give reasonable values for the stated conditions if the continuous beams and one-way slabs are part of a frame or continuous construction.
It should be noted that a continuous beam and one-way slabs should meet certain conditions otherwise this method cannot be used to analyze them. After the calculation of moments and shear, the continuous beam or one-ways slab can be easily designed using recommendations and specifications provided by ACI 318-19.
ACI Approximate Method
The ACI 318-19, section 6.2 contains approximate coefficients for calculating shears and moments, which can be used when the following conditions are satisfied:
- There are two or more spans.
- Spans are approximately equal, with the longer of two adjacent spans not greater than the shorter by more than 20 percent.
- Loads are uniformly distributed.
- The unfactored live load does not exceed 3 times the unfactored dead load.
- Members are prismatic.
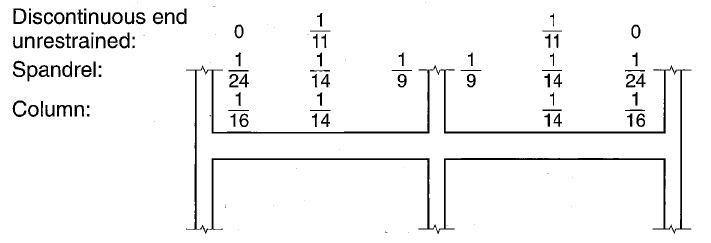
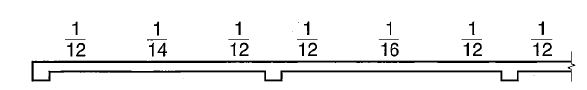
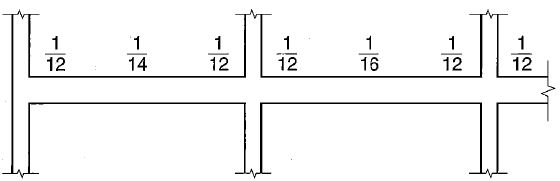
The coefficients by which approximate moments and shears are found is presented in Table-1. The same coefficients are presented in Figure-3 to Figure-6.
Table 1: Approximate moments and shears for non-prestressed continuous beams and one-way slabs
| Positive moment | ||
| Locations1 | Conditions2 | Mu |
| End span | Discontinuous end integral with support | wu ?n2/14 |
| End span | Discontinuous end unrestrained | wu ?n2/11 |
| Interior spans | All | wu ?n2/16 |
| Negative moment | ||
| Locations | Conditions | Mu |
| Interior face of exterior support | Member built integrally with supporting spandrel beam | wu ?n2//24 |
| Interior face of exterior support | Member built integrally with supporting column | wu ?n2/16 |
| Exterior face of first interior support | Two spans | wu ?n2/9 |
| Exterior face of first interior support | More than two spans | wu ?n2/10 |
| Face of other supports | All | wu ?n2/11 |
| Face of all supports satisfying (a) or (b) | (a) slabs with spans not exceeding 3 m (b) beams where the ratio of the sum of column stiffnesses to beam stiffness exceeds 8 at each end of a span | wu ?n2/12 |
| Shears | ||
| Locations | Conditions | Vu |
| Exterior face of first interior support | - | 1.15wu?n/2 |
| Face of all other supports | - | wu?n/2 |
1, 2: locations and conditions of continuous beams and non-prestressed one-way slab is explained in Figure-1.

Where:
?n: length of clear span measured face to face of supports for positive moments and the average of the two adjacent clear spans for negative moments, Figure-2.
wu: factored load per unit length of beam.


After the moments and shears are found, cross-sections and required reinforcements can be calculated. Beams are commonly cast monolithically with slabs. That is why they may be designed as T-section at mid spans on which positive moments act. As far as critical sections for negative moments area concerned, they should be designed as rectangular beams since the upper part of the beam is under tension and cracks.
Please click the following links for detailed design procedures of rectangular beams, doubly reinforced concrete beams, T-beams, and one-way slabs:
Design of Rectangular Reinforced Concrete Beam
Design of Doubly Reinforced Concrete Rectangular Beams with Example
Design Procedure of Reinforced Concrete T-beam with Example
How to Design Reinforced Concrete one-way Slab as per ACI 318-19? Example Included
FAQs
The ACI approximate method, which is also called the simplified method of analysis, is presented by ACI 318-19, section 6.5 to analyze continuous beams and non-prestressed one-way slabs. It is derived by elastic analysis, considering alternative placement of live load to produce maximum positive or negative moment at critical sections.
The approximate moments and shears, found by using ACI simplified method, give reasonable values for the stated conditions if the continuous beams and one-way slabs are part of a frame or continuous construction.
1. There are two or more spans.
2. Spans are approximately equal, with the longer of two adjacent spans not greater than the shorter by more than 20 percent.
3. Loads are uniformly distributed.
4. The unfactored live load does not exceed 3 times the unfactored dead load.
5. Members are prismatic.
A continuous beam is a beam that is loaded and has more than two supports. It can withstand greater loads by providing greater bending resistance along the length of the beam.
Read More
Thumb Rules and Specifications for Design of Reinforced Concrete Beams